7.01 Covering spaces
Below the video you will find accompanying notes and some pre-class questions.
- Next video: 7.02 Path-lifting, monodromy.
- Index of all lectures.
Notes
Intuition for covering spaces
(0.30) Take C∖{0} and consider the map
p:C∖{0}→C∖{0} given
by p(z)=z2. This map is surjective and 2-to-1. Nonetheless, we
do have something like an inverse locally: the square root function
(we actually have two local inverses differing by a sign
±√).
(4.00) We could equally have taken a branch cut
{z∈C∖{0} : Im(z)=0, Re(z)>0} and we
would have obtained two different local inverses for p. The point
of taking a branch cut is that C∖{0} is not
simply-connected but C∖B is simply-connected,
which is what lets us define an inverse on the complement of the
branch-cut.
(2.26) More precisely, if we excise a half-line B={z∈C∖{0} : Im(z)=0, Re(z)<0} then on C∖B we have two functions q+,q−:C∖B→C∖{0}
such that p(q±(z))=z. In this example, q−=−q+,
e.g.q+(1)=1, q−(1)=−1.
(5.11) Take f:C↦C∖{0},
f(z)=eiz. This map is surjective and ∞-to-1 (for
example f−1(1)={2πn : n∈Z}). On the
complement of a branch cut B we have infinitely many
well-defined local inverses qn=2πn−ilog):C∖B→C satisfying
qn(1)=2πn and p(qn(z))=z (that is exp(i(2πn−ilogz))=z). [The video is missing a factor of −i.]
(9.00) Roughly speaking, a covering map is a map p:Y→X
which is N-to-1 for some N and which has N locally-defined
inverses qn defined on simply-connected subsets of the target
(satisfying p∘qn=id).
(10.40) Notice that in the second example, the domain of the
covering map is C, which is simply-connnected, and the
local inverses qn are indexed by the integers
Z. This is, roughly speaking, how you deduce that the
target of the covering map has π1≅Z.
Definition
(11.20) Let p:Y→X be a continuous map.
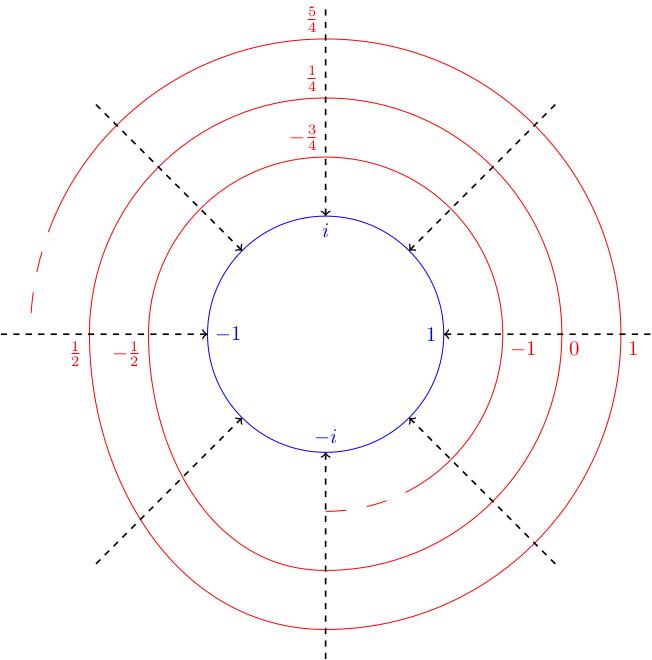
(18.36) Here is a picture of the exponential map p(z)=eiz
restricted to the real axis (so its image is the unit circle in
C):
- A subset U⊂X is called an elementary
neighbourhood if there is a discrete set F and a
homeomorphism h:p−1(U)→U×F such that
pr1∘h=p|p−1(U) (where pr1:U×F→U is the projection to the first factor).
- (13.30) You should think of an elementary neighbourhood as playing the role of the complement of a branch cut in our earlier examples: U is the subset on which you have locally-defined inverses to p.
- (13.57) The locally-defined inverses are parametrised by the discrete set F; in our examples we had F={−1,1} and F=Z. For each point m∈F we get a map qm:U→U×F→p−1(U) defined by qm(x)=h−1(x,m) which satisfies p(qm(x))=pr1(h(h−1(x,m)))=x.
- (16.07) We say p is a covering map if X is
covered by elementary neighbourhoods (i.e. every point x∈X
is contained in some elementary neighbourhood).
- Note that the word cover can now mean two things: covering map and open cover (like in the definition of compactness). Hopefully it will be clear what is meant from the context.
- (17.40) We say that a subset V⊂Y is an
elementary sheet if it is path-connected and p(V) is an
elementary neighbourhood.


More examples
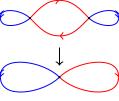
(24.05) Consider the figure 8, with its two loops drawn in red and
blue. The following picture defines a 2-to-1 covering space of the
figure 8:
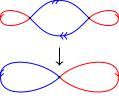
(26.22) Another covering space of the figure 8 is given by this
picture:
Pre-class questions
- Are there any other 2-to-1 covers of the figure 8? Remember, in each
case, the cross-point has two preimages which look like
cross-points, each edge has two preimages which connect the
cross-points.
Navigation
- Next video: 7.02 Path-lifting, monodromy.
- Index of all lectures.