1.08 Brouwer's fixed point theorem

Below the video you will find accompanying notes and some pre-class questions.

Previous video: 1.07 Induced maps.
Next video: 1.09 Homotopy equivalence.
Index of all lectures.

Notes

Brouwer's fixed point theorem

(0.30) Let

$F\colon D^2\to D^2$ be a continuous map, where

$D^2=\{(x,y)\in\mathbf{R}^2\ :\ x^2+y^2\leq 1\}$ is the 2-dimensional disc. Then there exists a point

$x\in D^2$ such that

$F(x)=x$ (a fixed point).

(1.40) Assume, for a contradiction, that

$F(x)\neq x$ for all

$x\in D^2$ . Then we can define a map

$G\colon D^2\to\partial D^2$ (where

$\partial D^2$ denotes the boundary circle) as follows: consider the ray starting at

$F(x)$ , passing through

$x$ and let

$G(x)$ to be the unique point of intersection of this ray with

$\partial D^2$ .

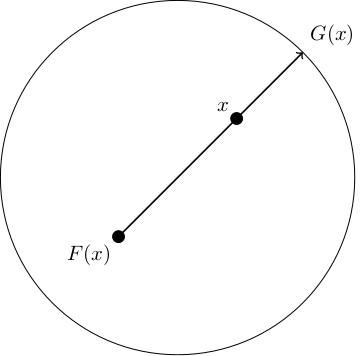
(3.45) If there were a fixed point, we would not know which ray to draw (as $F(x)=x$ ), so this map is only well-defined because we have assumed there are no fixed points.

(3.58) We claim that:

$G$ is continuous; this is intuitively clear, but requires proof (below).
$G(x)=x$ if $x\in\partial D^2$ ; this is clear, because if $x\in\partial D^2$ , no matter where $F(x)$ is, the ray from $F(x)$ to $x$ intersects the boundary at $x$ , so $G(x)=x$ .

(5.25) Given these two claims, we get a contradiction as follows. Let

$i\colon\partial D^2\to D^2$ be the inclusion map of the boundary; the composition

$G\circ i$ equals the identity map on

$\partial D^2$ by claim 2. This implies

$id=G_*\circ i_*\colon\pi_1(\partial D^2)\to\pi_1(\partial D^2).$ We have

$\pi_1(\partial D^2)=\mathbf{Z}$ , and this is saying that the identity map on the integers factors through the map

$i_*\colon\pi_1(\partial D^2)\to\pi_1(D^2)=0$ :

(7.30) This implies that

$G_*\circ i_*=0$ . This is impossible, as it implies

$n=G_*(i_*(n))=G_*(0)=0$ for all

$n\in\mathbf{Z}$ . Therefore we have a contradiction, and we have proved Brouwer's fixed point theorem, modulo claim 1.

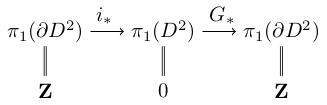
(9.20) It remains to prove claim 1 (that $G$ is continuous). We can write $G$ as a composition $H\circ j$ where:

$j\colon D^2\to D^2\times D^2\setminus\{(x,x)\ :\ x\in D^2\}$ is the map $j(x)=(x,F(x))$ .
(11.30) $H\colon D^2\times D^2\setminus\{(x,x)\ :\ x\in D^2\}$ is the map defined as follows. Given two distinct points $x\neq y$ in $D^2$ , let $H(x,y)$ denote the point where the ray from $y$ through $x$ intersects $\partial D^2$ .

(12.25) By definition, we have

$G(x)=H(x,F(x))=H(j(x))$ . By the properties of the product topology, the map

$j$ is continuous (more generally, if

$p$ and

$q$ are continuous then

$(p,q)$ is continuous). It therefore suffices to show that

$H$ is continuous.

(13.25) We can write $H$ explicitly in coordinates. The ray from $y$ through $x$ is given in parametric form by $y+t(x-y)$ . The condition that the ray meets the boundary of the disc is

$|y+t(x-y)|^2=1,$ which is a quadratic equation in

$t$ :

$t^2|x-y|^2+2t(x-y)\cdot y+|y|^2-1=0.$ (Note that the leading term is never zero since

$x\neq y$ ). This has solution

$t_\pm=\frac{-2(x-y)\cdot y\pm\sqrt{4((x-y)\cdot y)^2-4|x-y|^2(|y|^2-1)}}{2|x-y|^2}$ We are only interested in solutions with

$t\geq 0$ as the ray only points in one direction. Therefore the point

$H(x,y)$ is

$y+t_+(x-y)$ , which is now expressed purely in terms of rational functions and surds, all of which are continuous.

Pre-class questions

Brouwer's fixed point theorem tells us that continuous maps between 2-discs have fixed points. Is the same true for maps between 2-dimensional annuli? (An annulus is $S^1\times[0,1]$ ).
Brouwer's fixed point theorem also holds for maps $F\colon D^n\to D^n$ where $D^n$ is the $n$ -dimensional disc; can the proof above be adapted to cover this case, or are new ideas required?

Navigation

Previous video: 1.07 Induced maps.
Next video: 1.09 Homotopy equivalence.
Index of all lectures.