2.02 Bases, metric and product topologies
Below the video you will find accompanying notes and some pre-class questions.
- Previous video: Topological spaces, continuous maps.
- Next video: Subspace topology.
- Index of all lectures.
Notes
(0.00) In the last section, we defined topological spaces. In this section, I will tell you how you might specify a topology on a set. The idea comes from the theory of metric spaces.
Bases and the metric topology
(0.25) Given a metric space (X,d), how do I define a topology on X? Let B={Br(x) : x∈X, r≥0} be the set of all (open) metric balls in X. Let T={arbitrary unions of balls in B}.
X is in T because for any x∈X the ball B1(x) contains x, so X equals the union ⋃x∈XB1(x), which is in T by definition (it is a union of sets from B).
Unions of sets in T are unions of unions of sets in B, so in particular they are unions of sets in B and therefore in T. So T is closed under taking union.
(4.06) All that remains is to show that if U,V∈T then U∩V∈T.
Given a point x∈U∩V, we need to show that x is contained in a ball Bϵx(x)∈B such that Bϵx(x)⊂U∩V: then we have U∩V=⋃x∈U∩VBϵx(x) which is in T by definition. Since U∈T and x∈U we know that there is some ball Bs(u)∈B with x∈Bs(u)⊂U. Similarly, there is a ball Bt(v)∈B with x∈Bt(v)⊂V.
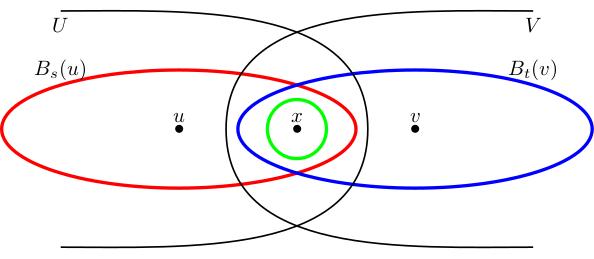
(6.22) We need to check that there is some radius ϵx such that Bϵx(x)⊂Bs(u)∩Bt(v).
Let ϵ1=s−d(u,x). I claim that Bϵ1(x)⊂Bs(u): if w∈Bϵ1(x) then d(w,u)≤d(w,x)+d(x,u)<ϵ1+d(u,x)=s, so w∈Bs(u).
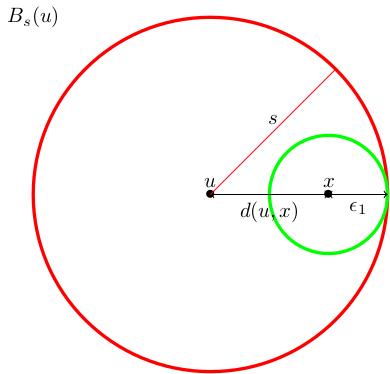
(8.55) Similarly, if ϵ2=t−d(x,v) then Bϵ2(x)⊂Bt(v). Set ϵx=min(ϵ1,ϵ2); then Bϵx(x)⊂Bs(u)∩Bt(v) as required.
- for all x∈X there exists b∈B such that x∈b (this will imply X∈T);
- for all U,V∈B and x∈U∩V there exists b∈B such that x∈b⊂U∩V (this will imply that T is closed under taking finite intersections).
Base for the product topology

- (16.10) For all (x,y)∈X×Y there exist U∈S such that x∈U and V∈T such that y∈V, so (x,y)∈U×V∈B.
- (17.08) Given U1×V1 and U2×V2, the
intersection is (U1×V1)∩(U2×V2)=(U1∩U2)×(V1∩V2).
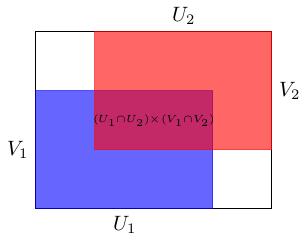
This implies that B is a base.
Pre-class questions
- In this video, instead of checking that all finite intersections of open sets are open, we checked that intersections of two open sets are open. Why is this sufficient?
- Let X be a set. Can you give a base for the discrete topology on
X? What is the smallest base you could give?
Navigation
- Previous video: Topological spaces, continuous maps.
- Next video: Subspace topology.
- Index of all lectures.