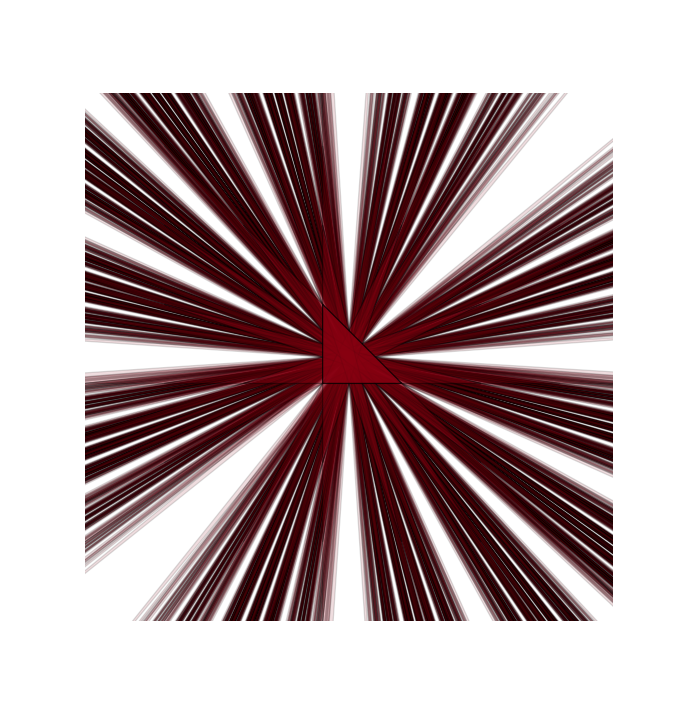
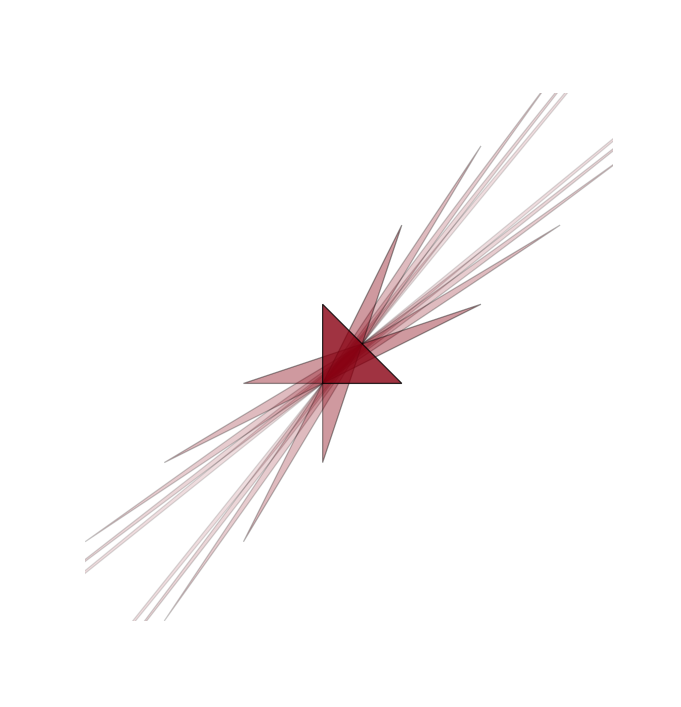
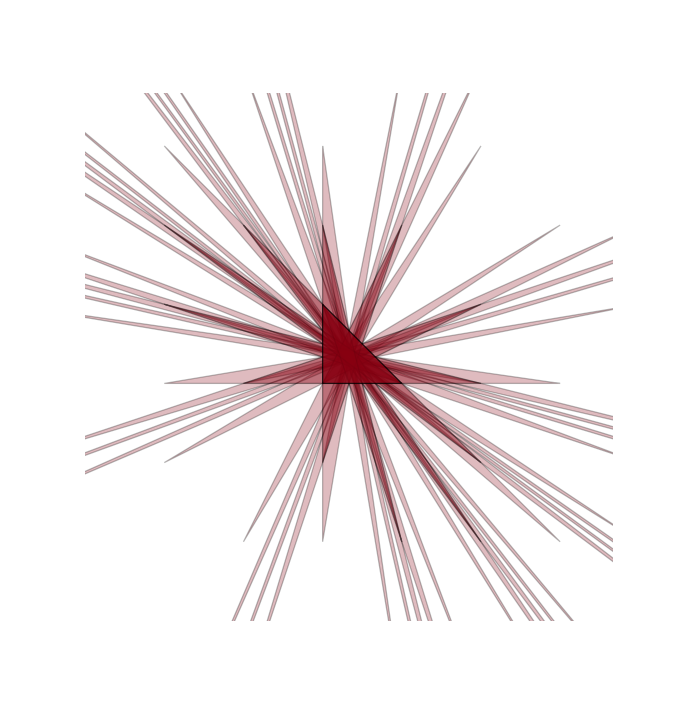
Developing map

Following on from my last post, here are more figures that will appear in my lectures on Lagrangian torus fibrations. This time, we see the image of the developing map for the integral affine structure for the base of a Lagrangian torus fibration on \(\mathbf{CP}^2\) obtained from the standard ``moment triangle'' by (a) one, (b) two, and (c) three nodal trades. The result is the union of all mutations of the moment triangle, which gives a rather lovely ``integral affine fractal'' in the plane. For me, mutations always seemed a little mysterious until I thought of drawing a picture like this. The point is that action coordinates are not globally defined on the base of a Lagrangian torus fibration, they only really make sense on its universal cover. When you look at two polygons related by a mutation, you're really looking at the image of two neighbouring fundamental domains in the universal cover under action coordinates. In the diagrams, the opacity decreases for fundamental domains which are far from the standard moment triangle (``far'' with respect to the word metric on the fundamental group and the obvious set of generators).

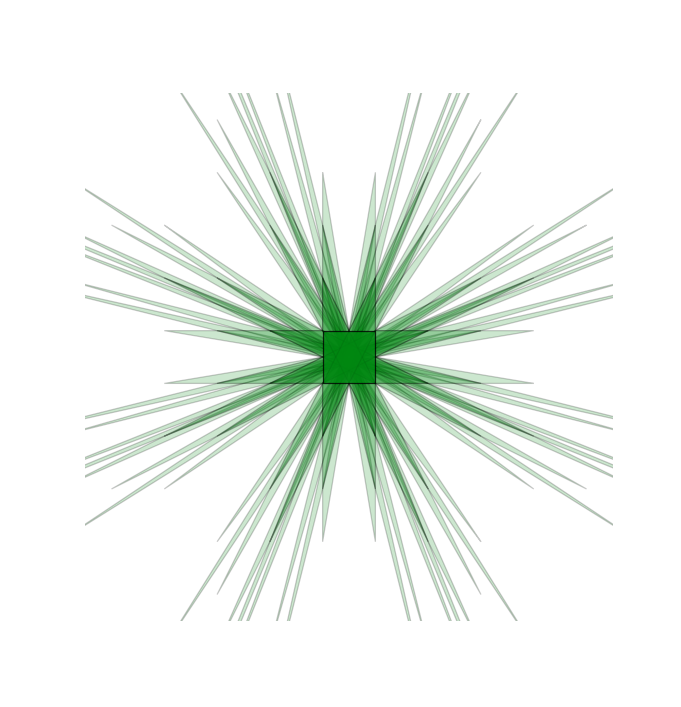
Finally, we see something similar for \(S^2\times S^2\) with four nodal trades:

Just for fun, here is one of the three-nodal trade fibration on \(\mathbf{CP}^2\) but going much deeper into the mutation tree.