sl(2,C) subalgebras, 1

Review of SU(3)

0.00 When we studied the representation theory of $G=SU(3)$ (or of its Lie algebra $\mathfrak{g}=\mathfrak{sl}(3,\mathbf{C})$ , one of the key pictures we studied was the root diagram: the weight diagram of its adjoint representation. This was a hexagonal configuration of roots at $L_{i}-L_{j}$ with $i,j\in\{1,2,3\}$ , $i\neq j$ .

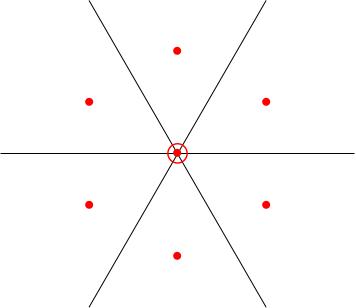
0.40 We'll write $\mathfrak{g}_{L_{i}-L_{j}}=\mathbf{C}\cdot E_{ij}$ for the root space corresponding to the root $L_{i}-L_{j}$ and $\mathfrak{g}_{0}$ for the weight space corresponding to $0$ . Recall that $\mathfrak{g}_{0}=\mathbf{C}\cdot H_{13}\oplus\mathbf{C}\cdot H_{23}=\left\{% \begin{pmatrix}\theta_{1}&&\\ &\theta_{2}&\\ &&-\theta_{1}-\theta_{2}\end{pmatrix}\ :\ \theta_{1},\theta_{2}\in\mathbf{C}% \right\}.$

1.20 This Lie algebra has the nice property that if we pick one root $L_{i}-L_{j}$ and its opposite root $L_{j}-L_{i}$ ), and we pick generators $E_{ij}\in\mathfrak{g}_{L_{i}-L_{j}}$ and $E_{ji}\in\mathfrak{g}_{L_{j}-L_{i}}$ then the three elements $E_{ij},\quad E_{ji},\quad H_{ij}=[E_{ij},E_{ji}]$ span a Lie subalgebra isomorphic to $\mathfrak{sl}(2,\mathbf{C})$ . Our study of the structure of $\mathfrak{sl}(3,\mathbf{C})$ representations used the three $\mathfrak{sl}(2,\mathbf{C})$ subalgebras we obtained this way: for example, it gave us the Weyl symmetry group. This will generalise.

Roots in general

The setup

3.05 Suppose $K$ is a compact matrix group with Lie algebra $\mathfrak{k}$ . Let's write $\mathfrak{g}$ for the complexification $\mathfrak{k}\otimes\mathbf{C}$ . Inside $K$ we have a maximal torus $T$ with Lie algebra $\mathfrak{t}$ . Let's write $\mathfrak{h}=\mathfrak{t}\otimes\mathbf{C}\subset\mathfrak{g}$ . The adjoint representation is a map $\mathrm{Ad}\colon K\to GL(\mathfrak{k})$ , defined by $g\mapsto\mathrm{Ad}_{g},\quad\mathrm{Ad}_{g}(X)=gXg^{-1}.$ The same formula defines a complex representation, also written $\mathrm{Ad}\colon K\to GL(\mathfrak{g})$ , if we allow $X$ to live in $\mathfrak{g}$ . By taking the derivative we get the Lie algebra representation $\mathrm{ad}\colon\mathfrak{k}\to\mathfrak{gl}(\mathfrak{g})$ and its complexification $\mathrm{ad}^{\mathbf{C}}\colon\mathfrak{g}\to\mathfrak{gl}(\mathfrak{g}).$

The root diagram

5.10 Because we have a maximal torus, we get a weight space decomposition $\mathfrak{g}=\bigoplus\mathfrak{g}_{\alpha}$ where $\mathfrak{g}_{\alpha}=\{X\in\mathfrak{g}\ :\ \mathrm{ad}_{H}X=\alpha(H)X\ % \forall H\in\mathfrak{h}\}.$ The direct sum is happening over a finite set of weights. Which weights occur in this direct sum?

6.00 $\alpha=0$ occurs, in other words $\mathfrak{g}_{0}\neq 0$ . This is because $\mathfrak{h}$ is an abelian Lie algebra, so $\mathrm{ad}_{H}(H^{\prime})=0$ for all $H,H^{\prime}\in\mathfrak{h}$ . Therefore $\mathfrak{h}\subset\mathfrak{g}_{0}$ (just like in the $SU(3)$ example).

Lemma:

7.00 In fact, $\mathfrak{h}=\mathfrak{g}_{0}$ .

Proof:

Suppose $Z\in\mathfrak{g}_{0}$ , i.e. $Z\in\mathfrak{g}$ and $\mathrm{ad}_{H}Z=0$ for all $H\in\mathfrak{h}$ . Then $[H,Z]=0$ for all $H\in\mathfrak{h}$ . In particular, $[H,Z]=0$ for all $H\in\mathfrak{t}\subset\mathfrak{h}$ .

8.15 Let's write $Z=X+iY$ where $X,Y\in\mathfrak{k}$ (so they are the real and imaginary parts of $Z$ ). Then $0=[H,Z]=[H,X]+i[H,Y]$ for all $H\in\mathfrak{t}$ , therefore both real and imaginary parts must vanish, and $[H,X]=[H,Y]=0$ . This means that $X,Y\in\mathfrak{k}$ commute with all elements in $\mathfrak{t}$ .

9.35 If either $X$ or $Y$ is not contained in $\mathfrak{t}$ then either $\mathfrak{t}\oplus\mathbf{C}\cdot X$ or $\mathfrak{t}\oplus\mathbf{C}\cdot Y$ will be an abelian subalgebra $\mathfrak{t}^{\prime}$ strictly containing $\mathfrak{t}$ . Then $\overline{\exp(\mathfrak{t}^{\prime})}$ is a torus strictly containing $T$ , which contradicts the assumption that $T$ is a maximal torus.

11.00 Which other weights occur?

Definition:

Any nonzero weight of the adjoint representation is called a root. (The weight diagram is called a root diagram, the weight vectors are called root vectors, etc). We will write $R$ for the set of roots.

Action of root vectors

Lemma:

11.45 If $X\in\mathfrak{g}_{\alpha}$ and $Y\in\mathfrak{g}_{\beta}$ then $[X,Y]\in\mathfrak{g}_{\alpha+\beta}$ . (We won't prove this: it's an exercise, very similar to the corresponding results for $\mathfrak{sl}(2,\mathbf{C})$ and $\mathfrak{sl}(3,\mathbf{C})$ , like " $X$ moves things to the right and $Y$ moves things to the left".)
13.15 If $X\in\mathfrak{g}_{\alpha}$ and $Y\in\mathfrak{g}_{\beta}$ then $K(X,Y)=0$ unless $\alpha+\beta=0$ .
If $\mathfrak{g}$ is semisimple then $\alpha\in R$ if and only if $-\alpha\in R$ .

Proof:

14.00 (2). Pick a basis of $\mathfrak{g}$ consisting of root vectors. We'll compute the matrix of $\mathrm{ad}_{X}\mathrm{ad}_{Y}$ with respect to this basis and then take the trace to find $K(X,Y)$ . Suppose $Z\in\mathfrak{g}_{\gamma}$ is one of our basis vectors. Where does $Z$ go under $\mathrm{ad}_{X}\mathrm{ad}_{Y}$ ? By part (a), $\mathrm{ad}_{X}\mathrm{ad}_{Y}(Z)=[X,[Y,Z]]\in\mathfrak{g}_{\alpha+\beta+\gamma}$ .

15.25 Let's write $\mathrm{ad}_{X}\mathrm{ad}_{Y}$ as a block matrix with respect to the splitting into weight spaces (i.e. the $i, j$ block in the matrix is the matrix of the map $\mathfrak{g}_{\lambda_{i}}\to\mathfrak{g}_{\lambda_{j}}$ ).

15.55 The diagonal blocks encode the maps $\mathfrak{g}_{\lambda}\to\mathfrak{g}_{\lambda}$ . But if $\alpha+\beta\neq 0$ then $\mathrm{ad}_{X}\mathrm{ad}_{Y}$ sends $\mathfrak{g}_{\lambda}$ to $\mathfrak{g}_{\lambda+\alpha+\beta}\neq\mathfrak{g}_{\lambda}$ , so there are no nonzero diagonal blocks if $\alpha+\beta\neq 0$ . Therefore the trace of this matrix vanishes and $K(X,Y)=0$ .

16.40 (3) If $\mathfrak{g}$ is semisimple then the Killing form is nondegenerate, so for all nonzero $X$ there exists $Y$ such that $K(X,Y)\neq 0$ . In particular, if $X\in\mathfrak{g}_{\alpha}$ then there exists $Y$ such that $K(X,Y)\neq 0$ .

17.30 Take the components of $Y=\sum_{\lambda}Y_{\lambda}$ with respect to the weight space splitting. Then $K(X,Y_{\lambda})=0$ unless $\lambda=-\alpha$ . Therefore, if $K(X,Y)\neq 0$ , we must have $Y_{-\alpha}\neq 0$ , so $\mathfrak{g}_{-\alpha}\neq 0$ and $-\alpha\in R$ .

18.35 The trick for extracting the $\mathfrak{sl}(2,\mathbf{C})$ subalgebras will be the following theorem which we'll prove next time.

Theorem:

If $X\in\mathfrak{g}_{\alpha}$ , $Y\in\mathfrak{g}_{-\alpha}$ are nonzero then $X$ , $Y$ and $H=[X,Y]$ will span a subalgebra isomorphic to $\mathfrak{sl}(2,\mathbf{C})$ .

Pre-class exercise

Exercise:

True or false: If we use a sub-maximal torus, is it still true that $\mathfrak{h}=\mathfrak{g}_{0}$ ? If so, why? If not, what do we get instead?