Lemma:
The dihedral angle between two of the root hyperplanes in a root system can be 90, 60, 45 or 30 degrees and nothing else.
Classification of root systems, 2
Classification of root systems, continued
Dihedral angles
The proof of this lemma will appear in another video. Let's see some examples of root systems which have these dihedral angles.
Examples
These are the root systems of SU(2) times SU(2), SU(3), SO(5) and G_2 respectively.
For SU(2) times SU(2), the orthogonal projection of any root onto any of the other root lines gives zero (as the roots are all orthogonal to one another). Therefore n beta alpha equals 0 for all pairs of non-collinear roots alpha and beta. The angles between the mirrors are 90 degrees.
The example of SU(3) we've studied in some detail already. The dihedral angle is 60 degrees.
The example of SO(5) has some long roots and some short roots. For example, if we project onto the line through a short root then we get n beta alpha is minus 1, zero or 1. If we project onto a long root, we get n beta alpha is minus a half, 0 or a half. The minimal dihedral angle appearing here is 45 degrees.
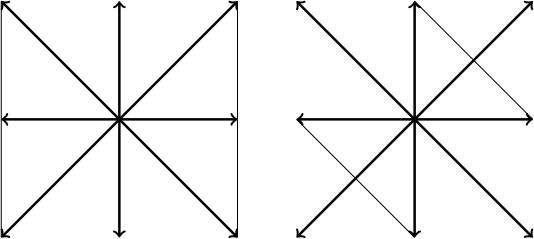
The final example comes from a group called G_2. This is one of the few groups we haven't met. There are six short roots and six long roots. The angle between neighbouring roots is 30 degrees. If you project from a short root beta to a long root alpha you end up halfway to the long root (so n beta alpha equals plus or minus a half) and if you project from the long to the short root you end up three halfths of the way along the root line (so n alpha beta equals plus or minus three halfths).
Remark:
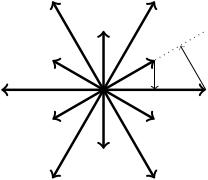
It will turn out that these four are the only 2-dimensional root systems.
Dynkin diagrams
Drawing pictures in 2-d and making models in 3-d is all very well, but what about root systems in higher dimensions? It turns out there's a very efficient way of encoding these diagrams using a graph called a Dynkin diagram or Coxeter-Dynkin diagram.
Definition:
Pick your favourite Weyl chamber. The boundary is a collection of n mirrors corresponding to simple roots. We draw a dot for each mirror. We connect the dots with:
-
no edges if the dihedral angle is 90 degrees,
-
one edge if the dihedral angle is 60 degrees,
-
two edges if the dihedral angle is 45 degrees,
-
three edges if the dihedral angle is 30 degrees.
We further decorate the diagram with arrows on edges going from the dot corresponding to a short root to the dot corresponding to a long root.
Examples
Example:
For SU(2) times SU(2), there are two mirrors at 90 degrees, so the Dynkin diagram is just two dots, no edges.
Example:
For SU(3), the Weyl chamber is cut out by two mirrors at 60 degrees to one another, so the Dynkin diagram is two dots connected by an edge.
Example:
For SO(5), the Weyl chamber is cut out by two mirrors at 45 degrees. The Dynkin diagram is therefore two dots connected by two edges; the edges have arrows pointing from the mirror corresponding to the short root to the mirror corresponding to the long root (in this case, the diagram is symmetric, so it doesn't matter which direction we draw the arrows).
Example:
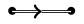
For G_2, the Weyl chamber is cut out by two mirrors at 30 degrees to one another. The Dynkin diagram is therefore two dots with three edges between them (and arrows pointing from the short roots to the long roots).
Example:
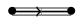
The 3-dimensional example we saw at the end of the last video had three mirrors making pairwise angles 60, 60 and 90 with one another. The Dynkin diagram is therefore three dots in a line, with single edges connecting the first to second and second to third dots.
Classification theorem
Theorem:
If R is the root system of a compact semisimple Lie group then the Dynkin diagram of R is a disjoint union of diagrams from the following list. (The diagrams on the list are connected; disjoint unions of Dynkin diagrams correspond to taking the product of the corresponding Lie algebras).
Remark:
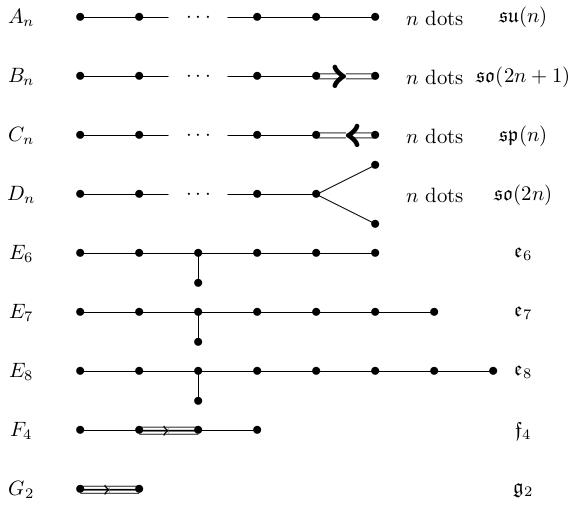
This is four infinite families of Dynkin diagrams A_n, B_n, C_n, D_n and five exceptional cases E_6, E_7, E_8, F_4, G_2. The four infinite families were known classically; the exceptional groups were discovered by people trying to classify them. They are hard to construct.
Remarks
Remark:
Remember that this is a classification of semisimple Lie algebras up to isomorphism: it's possible to have different groups with the same Lie algebra and hence the same Dynkin diagram.
Example:
The first infinite family is A_n. The subscript tells you the number of dots. These Dynkin diagrams are just chains of n dots, the first connected to the second, second to the third etc by single edges. These are the Dynkin diagrams of SU(n + 1).
Example:
The second infinite family is B_n. These are the Dynkin diagrams of the odd-dimensional rotation groups SO(2 n + 1). The diagram is similar to A_n except that the final two dots in the chain are connected by a double arrow pointing from vertex n minus 1 to vertex n, so the final dot corresponds to a longer simple root.
Example:
The family C_n is very similar except that the arrow goes the other way. These correspond to a family of groups called the symplectic groups S p n which we haven't discussed.
Example:
The family D_n has a chain of n minus 2 dots with two further dots sprouting off the last dot in the chain. All edges are single edges. This is the Dynkin diagram of SO(2 n) (even-dimensional rotation group).
Example:
The E_6, E_7, E_8 graphs are respectively 5, 6, 7 dots in a chain with one dot coming off the third dot (all edges single). This is not an infinite family: for example, the obvious thing you'd call an E_9 diagram doesn't correspond to a root system.
The F_4 diagram has four dots connected 1 to 2, 2 to 3 and 3 to 4 with 2 to 3 being a double arrow. G_2 has two dots connected by a triple arrow.
Remark:
It's quite surprising that we've been studying this big class of groups, but aside from one infinite family (symplectic groups) and five exceptional cases we'd never have dreamed of by ourselves, we're already familiar with all the examples.
Remark:
The Dynkin diagram is an amazingly economical way of encoding information. For example, the E_8 diagram is a complete recipe for building a configuration of eight 7-dimensional mirrors in 8-dimensional Euclidean space.
In what remains of the course, we will fill in some of the details of the proof of this theorem and the earlier lemmas.
Pre-class questions
Exercise:
Just by looking at the root diagrams, can you figure out the dimensions of the Lie algebras little s o 5 and little g_2?
Exercise:
Sketch the (3-dimensional) root system corresponding to your choice of Dynkin diagram from amongst: A_3, B_3, C_3 and D_3.